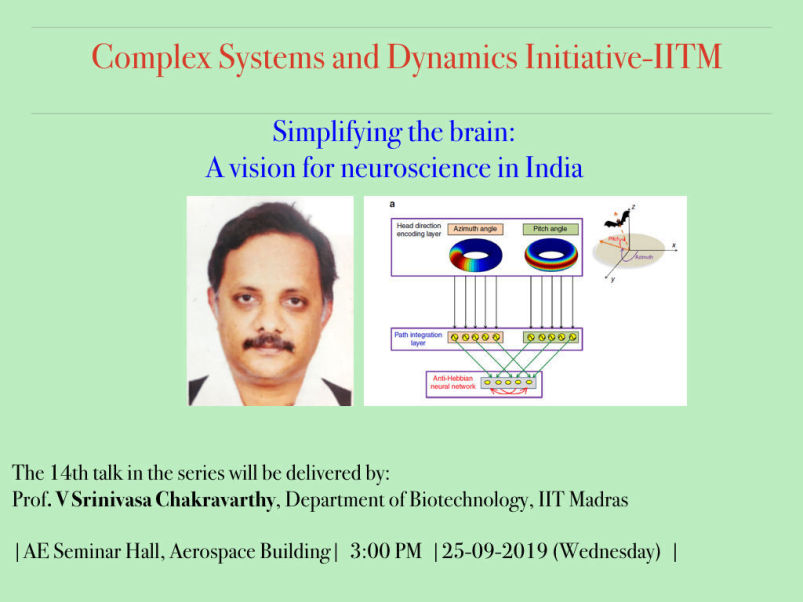
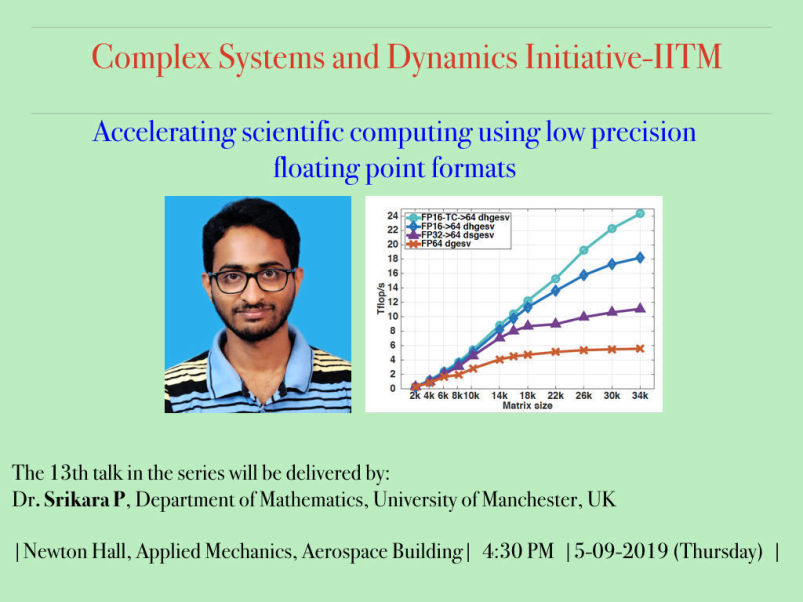
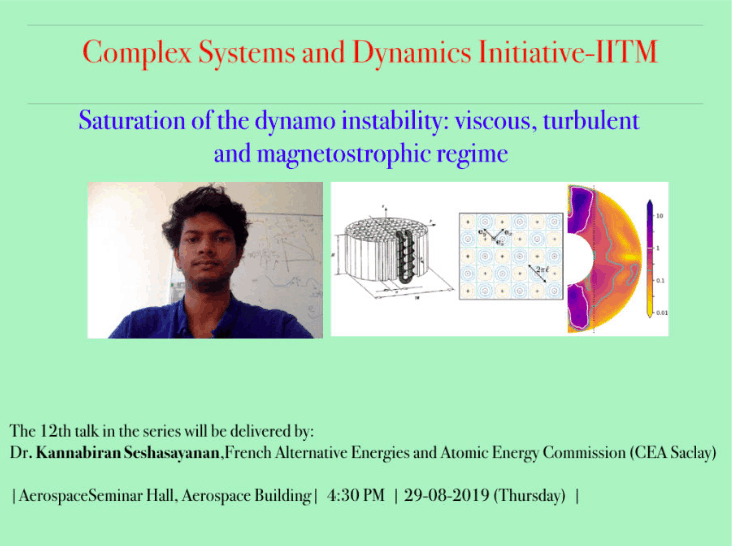
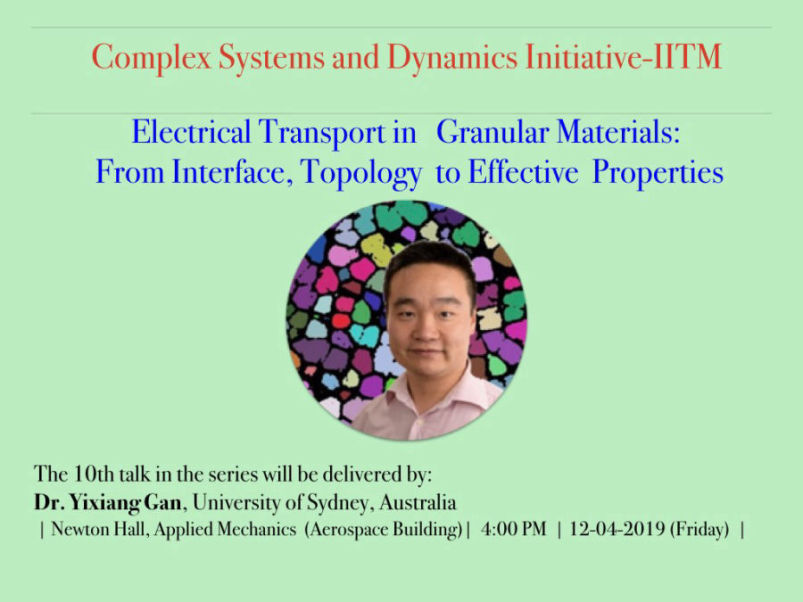
Prof Sarma L Rani

University of Alabama
USA

Mobirise

15th Seminar: October 3, 2019

On the Neumann boundary condition for the acoustic-wave Helmholtz Equation, and the relationship between pressure and density fluctuations 

Abstract
Acoustic wave propagation in a duct is governed by the Helmholtz equation which is derived from the linearized fluctuating forms of the mass momentum and energy balance equations. For 1-D domains the Helmholtz equation is a second-order ordinary differential equation (ODE) while the fluctuating balance equations are all first-order ODEs. As a result one needs two boundary conditions for the spatial pressure fluctuation p(x) in order to solve the Helmholtz equation while only one boundary condition each is needed for ρ u and p in case of the fluctuating balance equations. Accordingly this study was motivated by two prinicpal objectives. The first was to develop the exact Neumann (or derivative) boundary condition at the inlet to a quasi 1-D ductneeded to solve the Helmholtz equation. Such an exact boundary condition would ensure that the spatial pressure fluctuations p(x) obtained by solving the Helmholtz equation are identical to the p(x) obtained through the solution of the fluctuating balance equations. The second principal objective was to determine the exact relationship between the density and pressure fluctuations ρ and p respectively so that the ρ(x) calculated using the Helmholtz-equation p(x) is again identical to the ρ(x) obtained by solving the fluctuating balance equations. The exact ρ-p relation is also compared with the “classical” relation namely ρ = p/c^2 enabling us to evaluate the accuracy of the latter. The Neumann boundary conditions and the ρ-p relations were developed for five cases with axially uniform and non-uniform duct cross-sectional areas as well as homogeneous and inhomogeneous mean flow properties such as the velocity temperature density and pressure. It is seen that the ρ = p/c^2 relation is valid only for the cases with uniform cross-sectional area and homogeneous mean properties. For the cases with uniform cross-section inhomogeneous mean properties (with zero or uniform mean flow) the “classical” ρ relation suffers from significant errors both in amplitude and phase.


Publications

Prof V Srinivasa Chakravarthy

Department of Biotechnology 
IIT Madras, India

Mobirise

14th Seminar: September 25, 2019

Simplifying the brain: A vision for neuroscience in India

Abstract
Brain is often touted as “one of the most complex objects in the universe,” a perfectly unscientific statement considering our ignorance of the nature of “objects” found all over the universe. In this talk, the speaker argues that part of the reason behind this undue “complexification” of the brain lies in the profoundly descriptive traditions of biology. With the availability of sophisticated measurement tools, - and the big data revolution in the air, -currently there is movement to generate mountains of brain data without making a commensurate effort to develop elegant brain theories that can explain the data. By separating principles from details, engineers create and master complex systems. The brain is no different.

As a demonstration of how it is possible to develop simple brain theories/models that can explain diverse functions of brain systems, the speaker outlines his lab’s (CNS Lab) decade-long work in a brain system called the Basal Ganglia, a part of the brain associated with Parkinson’s disease. Next the speaker describes the CNS lab’s work on spatial navigation functions of another brain system called the hippocampus. Discovery of the “spatial cells” of the hippocampus was awarded the Nobel prize in 2014. The CNS lab had developed a simple model that can explain a wide variety of phenomena related to spatial navigation in 2d (in rats and mice) and in 3D (in bats).

Taking the above work to its logical consummation, the speaker outlines his lab’s plans to build a reduced model of the whole brain called the MESOBRAIN. The MESOBRAIN, once realized in software and hardware, is expected to have immense applications in medicine and engineering. 


More details

Dr. Srikara P

Department of Mathematics
University of Manchester, UK

Mobirise

13th Seminar: September 5, 2019

Accelerating scientific computing using low precision floating point formats  


Abstract
Motivated by developments in machine learning, hardware that support low precision floating-point formats are becoming increasingly available. These new hardware have enormous computational power and developing algorithms which can exploit them is of interest. In this talk a specific case of solution of linear system of equations, which has applications in almost all the branches of science and engineering is considered. First a Generalised Minimal Residual method-based iterative-refinement (GMRES-IR) algorithm for the solution of a linear system, that can exploit these low precision formats will be discussed, followed by its computational benefits.

Limited range of some of these low precision formats pose serious difficulty in applying GMRES-IR to matrices arising from actual applications. An algorithm to overcome this challenge will be discussed, and numerical experiments for matrices from applications such as structural mechanics will also be considered. Other potential application of these low precision formats in computational mechanics will also be discussed.


Presentation slides

Dr. Kannabiran Seshasayanan

CEA Saclay
France

Mobirise

12th Seminar: August 29, 2019

Saturation of the dynamo instability: viscous, turbulent and magnetostrophic regime 

Abstract
The dynamo instability explains the spontaneous generation of magnetic field by the flow of an electrically conducting fluid. This instability is believed to occur in planetary and stellar cores, where the conducting fluid is driven by convective and/or mechanical forces. A key challenge in dynamo theory is predicting the magnitude of the resulting magnetic field when the dynamo instability saturates. Analytical examples of dynamo instability give rise to a magnetic field at saturation to depend on the viscosity of the fluid. While in most astrophysical objects and in all laboratory experiments, the dynamo instability occurs over a highly turbulent background flow where the magnetic field is expected to be independent of the viscosity. Due to the high Reynolds number of the flow not much theoretical progress has been made while numerically, fully three dimensional turbulent simulations are quite expensive and do not reach high Reynolds numbers even with modern-day supercomputers.

In this talk I will illustrate an asymptotic reduced system of equations which when resolved numerically capture the turbulent scaling of the dynamo instability. Later I will introduce a simple analytical model that realise the high-Reynolds number scaling and also the magnetostrophic scaling regime where the magnetic field is governed by the global rotation rate.



Prof Ramakrishna Ramaswamy

IIT Delhi (formerly School of Physical Sciences, 
Jawaharlal Nehru University, India)

Mobirise

11th Seminar: April 23, 2019

On chance ("Chance ki baat")

Abstract
Chance is crucial in a diverse range of situations in the natural
world. To paraphrase Monod (1971), nature relies on chance and not on destiny. Depending on the context, chance can mean different things to different people. A chance event may either be a consequence of intrinsic fluctuations, or may be due to incomplete or imprecise knowledge. The notion of contingency is closely tied into that of chance, and thus uncovering the underpinnings - whether this is due to
underlying stochastic phenomena or underlying chaotic dynamics - is of great interest.

Using examples from dynamical systems theory, I will discuss the role of chance when there are several coexisting dynamical attractors, with attractor basins that are intermingled in a complex manner. Small uncertainties in determining the initial state can lead to very large uncertainties in the outcomes. Intrinsic noise, on the other hand, plays a major role in small systems where the dynamics is stochastic.

Both phenomena occur in biological systems and are exploited, at a systems level, in different ways. On the one hand, chance provides the possibility of complex dynamical states such as chimeras, and on the other, chance allows for stochastic switching both in the realm of dynamics within a cell or within a population.


Video

Prof Yixiang Gan

School of Civil Engineering,
University of Sydney, Australia

Mobirise

10th Seminar: April 12, 2019

Electrical Transport in Granular Materials: From Interface, Topology to Effective Properties

Abstract
Granular media, as a typical heterogeneous material, include everything from sand on the beach to flour and salt on our dining table, and can display some characteristics of gases, liquids and solids. Granular media are commonly used in energy systems to store, convert, capture and produce energy. Examples include lithium-ion batteries, nuclear materials, and thermal storage systems. This talk will discuss the fundamental mechanics of granular materials arising from the intrinsic multi-scale, multi-phase and multi-physics nature. Regarding electron transfer through the media, interfacial and topological structures are shown to play a significant role in determining the modes of transport. In this talk, we will present the transport phenomena in heterogenous media through a few specific examples, to demonstrate the granular origins of effective properties of these materials form the interfacial behaviour (contact mechanics) and topological features (complex network). 


Presentation slides

Prof Mahesh Panchagnula

Department of Applied Mechanics 
IIT Madras, India

Mobirise

9th Seminar: March 4, 2019

Dynamics of human crowd

Abstract
Gatherings of large numbers of people into confined spaces is a common occurrence in our country. We will discuss the various models used to understand the dynamics of such crowds as well as "empirical" data from video feed. We will discuss the two types of accidents that are common to dense crowds - stampedes and crushes - from a physics perspective. We show that the transition between orderly motion and a disorderly state is a non-equilibrium first order phase transition. In addition, we show that the transition is hysteretic whose width increases as the domain size increases. This gives rise to a possibility that crushes and stampedes (which occur primarily in the disorderly state) can be averted or reversed when both states are dynamically stable. In order to enforce this reversal, we will show that an optimal placement of "game-changers" (say, police) is possible from understanding the fluid dynamics of crowd motion in the orderly state.



Video

Prof Abhijit Chaudhuri

Department of Applied Mechanics
IIT Madras, India

Mobirise

8th Seminar: February 19, 2019

Interesting Researches on Complex Subsurface Systems Related to Energy Security and Protection of Environment 

Abstract
Subsurface hydro-geologic systems are heterogeneous and the evolutions of physical and chemical properties of the system by chemical reactions and mechanical damages are very complex. The evolutions get accelerated by various human intervention as the subsurface system is (i) the largest source of fresh water; (ii) an enormous source of energy (geothermal, oil & gas, and radio-active minerals); and (iii) a possibly the safe repository for contaminants (biodegradable), nuclear wastes and CO2. For long-term safety and reliability assessments of the extractions of resources from subsurface reservoirs and geological storage of wastes, we must consider modeling of multi-phase flow, reactive transport, heat transfer through porous medium as well as deformation of porous medium. These physical processes are strongly coupled. Flow instabilities due to large density and viscosity differences, permeability alteration by dissolution/precipitation of minerals, fracking, induced seismicity etc. are some of the interesting phenomena. Multi-scale heterogeneity not only contributes uncertainty but also results in drastic differences in evolution of patterns. In this presentation, I will discuss about our researches for past 10 years related to natural/engineered hydrothermal systems, CO2 sequestration, enhanced oil and gas recovery.



Presentation slides

Complex Systems & Dynamics     Indian Institute of Technology Madras     Chennai 600036     India